
If, furthermore, X is metrizable, then so is X/ M.

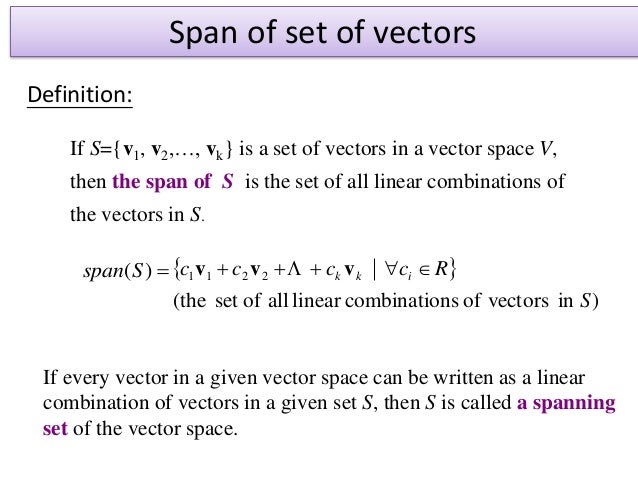
Then X/ M is a locally convex space, and the topology on it is the quotient topology. The mapping that associates to v ∈ V the equivalence class is known as the quotient map.Īlternatively phrased, the quotient space V / N These operations turn the quotient space V/ N into a vector space over K with N being the zero class. do not depend on the choice of representatives). It is not hard to check that these operations are well-defined (i.e. Scalar multiplication and addition are defined on the equivalence classes by The quotient space V/ N is then defined as V/~, the set of all equivalence classes induced by ~ on V. The equivalence class – or, in this case, the coset – of x is often denoted It is easy to check that S2 is closed under addition and scalar multiplication. Optimization and its applications: Much of machine learning is posed as an. A subset of R3 is a subspace if it is closed under addition and scalar multiplication.
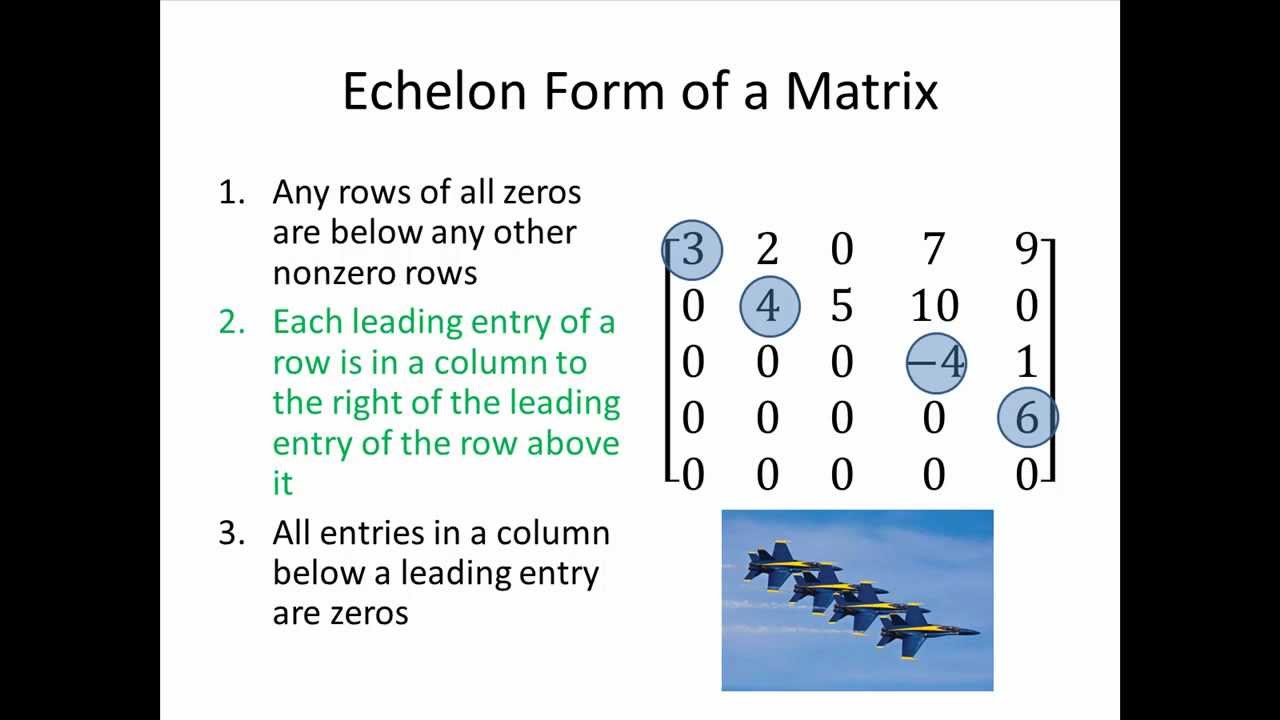
Linear algebra subspace definition how to#
The focus is clearly on the most relevant aspects of linear algebra for machine learning and to teach readers how to apply these concepts. From this definition, one can deduce that any element of N is related to the zero vector more precisely, all the vectors in N get mapped into the equivalence class of the zero vector. linear algebra methods with examples from machine learning differentiates this book from generic volumes on linear algebra. That is, x is related to y if one can be obtained from the other by adding an element of N.
We define an equivalence relation ~ on V by stating that x ~ y if x − y ∈ N. Let V be a vector space over a field K, and let N be a subspace of V. row equivalent matrices: Two matrices are row equivalent if one can be obtained from the other by a sequence of elementary row. Note: A theorem tells us that the two definitions of rank of a matrix are equivalent. 4.2 Generalization to locally convex spacesįormally, the construction is as follows. The rank of a linear transformation (and hence of any matrix regarded as a linear transformation) is the dimension of its range.4 Quotient of a Banach space by a subspace.


 0 kommentar(er)
0 kommentar(er)
